I am lucky that I work in a school where I am afforded ample opportunities to attend high quality professional development conferences, trainings, or seminars every year.  In regards to math, Marilyn Burns has to be my favorite rock star.  If I had my choice to spend an afternoon with her or Alan Jackson, I'd have to go with Marilyn.  I saw her for the first time when I weaseled my way into a math conference as a student teacher and every time I have seen her since has been just as fun and inspiring as the first time.  (Now I won't say how long ago it was that I first saw her, or how many times, that would just make both of us look old.)  But it was Marilyn Burns who taught me that you have to delve into the thought processes of a student if you truly want to learn how well they understand something.  I loved the idea of writing about math, you always need good topics for writing and math is an excellent one.  This idea also fits in beautifully with my mean, evil, step-teacher persona.  "What? the kids asked, "You mean we have to write during math?  You really ARE evil!"  Bwaaa ha ha! Oh yes...I am!  

So, my students write about math constantly.  Not only do they do it for assessments, they do it for problem solving.  When we problem solve in math class, true problem solving, I don't check work or answers.  Students have to work like real mathematicians, solving the problem in multiple different ways, and checking their results.  They then have "math conferences" where they circulate the room and compare strategies and results with their fellow mathematicians.  They then have to make connections, just like we do in reading; problem to self, problem to problem, problem to world.  Sometimes we even do First, Next, Then, Last books about the problem solving process.  Sequencing doesn't only happen in language arts folks.  This is how scientists and mathematicians do it, so this is how we do it!

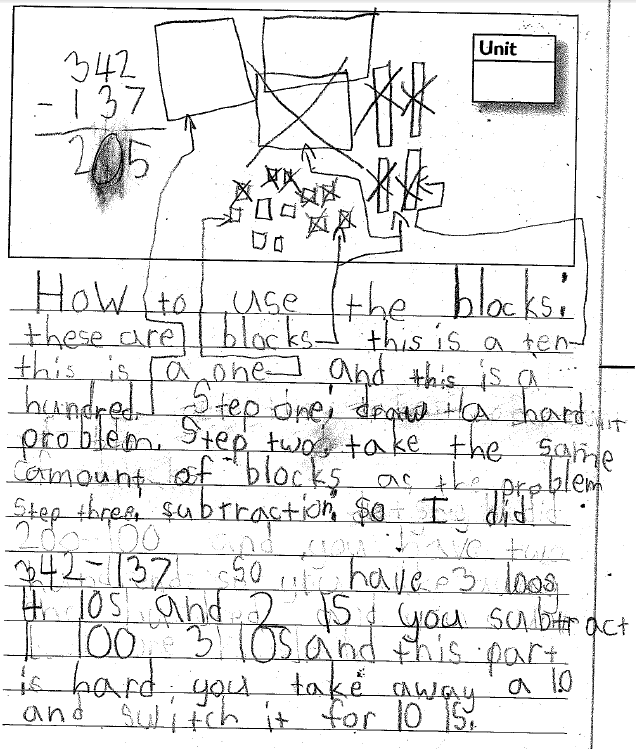
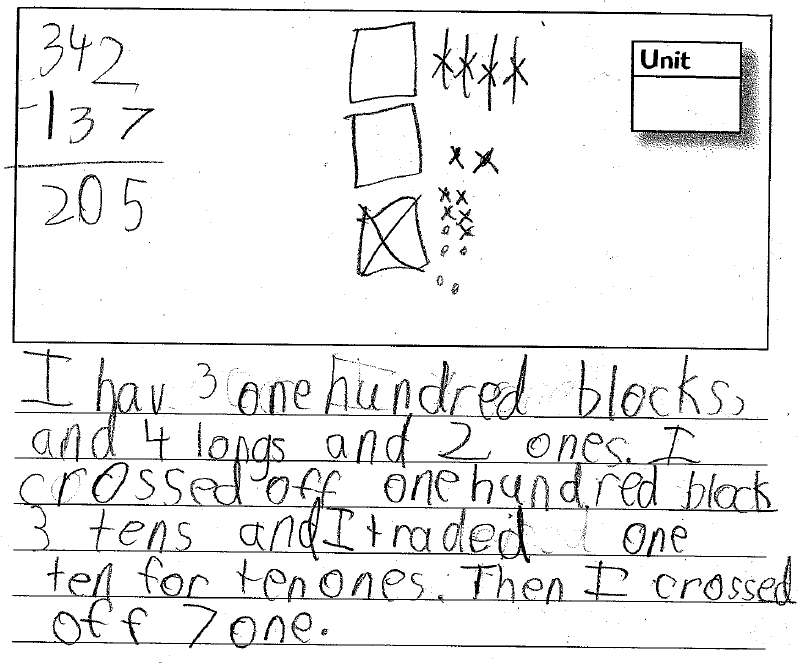
Now, if you look at the few samples I have below you can clearly see the differences in understanding, even among the three who got the correct answer.  With information like this I have a spot-on accurate assessment of their abilities.  I will also know when someone gets an answer right on accident.  I had a student explain that 4/5 was more than 3/4 because 4+5 was more than 3+4.  Without that explanation this answer would have been counter productive for me as the teacher.  I've also had students blow me away by creating algorithms that they had never been taught.  One student, with a similar problem to the one below explained the 2-7 section of the problem as being a -5, which he subtracted from what he got in the rest of the algorithm.  Holy cow!  Now that's a kid with some number sense.

So if these kids now take a common core assessment, what is new for them?  Certainly not explaining their thinking or providing evidence.  This process of explaining your computation and reasoning is old hat for them.  So I say to Common Core, bring it!  My students first graders will be ready.

Even if I wasn't using computers and iPads in my classroom, my teaching would still be completely different from what it was five to ten years ago.  Way back when, I started learning about teaching for true understanding, critical analysis, and creative creation.  These ideas was alluded to in my teacher training, but that was so long ago they were just in their infancy for most of the teaching community.  Working with manipulatives and understanding the concepts and patterns of math BEFORE showing how to record them with arithmetic algorithms was just the first step.  Now I ask students to use what they know to solve problems well above their grade level using teamwork and critical thinking skills.  Being smart is using what you know to figure out what you don't know.  Even after this arduous process I won't check students' work because that's not how it works in the real world.  Mathematicians and scientists don't have anyone to tell them if their theories and calculations are correct.  They compare results with their peers, so that is what my students do.  Storytelling has changed as well.  No longer do I accept just regular writing.  I want exciting and precise vocabulary, proper organization, and exactness of detail.  And the excuse, but they are just first graders won't fly with me.  They aren't JUST first graders, they are FIRST GRADERS!  Empower the students, teach them foundational skills, get out of their way, and they will amaze you.  Now, add in to this the use of computers and iPads and the revolution grows.  Geography takes on true meaning when you are in the midst of a mystery location call, bloggers have a world wide audience, your partner on that last project was not your buddy from class but your buddy from across the world.  My students have close to the sum of human knowledge at their fingertips.  But as Harold Wheeler says, they need to develop their crap detectors, because let's face it, there is a lot of crap on the internet.  My kiddos don't me to teach them details, they need me to teach them how to find the accurate information they need in order to solve problems.  If you can be replaced by a video, you should be!  So, with the switch to the "new" common core I wonder what is "new" about any of it.   Assessing the processing of information, critical thinking, and problem solving skills of students is nothing new at all.  It is just so much more exciting and customizable with technology.

Sometimes you just need to get away from it all!  Well then, just go to Fiji and relax on the beach.  That's what our first graders will do.  This past week we started to create Fiji in the back office.  This is a place where the students (and teachers) can go when they need a break or a cooling off period.  We started by painting the water, the sandy beach, and the palm trees.  I even added a plastic coconut "drink." (You can see it on the desk.)  But we aren't finished yet.  I'm going to bring in a beach towel, Ms. Shapp said she had an old beach chair we could add, and we are hoping for a grass skirt donation to put around the desk.  The kicker would be if we could get a small blow-up boat, but we'll have to do some "fishing around" for that.  So far Fiji has been entirely too popular, but that always happens when our get-away spot is new.  Wouldn't you be there all the time?  In a few days in won't be so novel and will be used as intended.  In the past I've had Antarctica as a place to "cool off" with penguins, and a field with a stuffed lamb friend.  But one word of warning, be sure to put it in your sub plans.  A few years ago a sub in my friend's second grade class was not amused when a student disappeared and then informed her he had been to Hawaii.

Are you cringing already?  I know, many adults say they "aren't math people."  But you are wrong.  You might not be fast at your reasoning and calculating, but that doesn't mean you can solve mathematical problems logically.  Really, anyone can do it.  My first graders are proof.  What was this problem they worked on for so long you wonder.  Well, here's the best part, some of the kiddos needed more time the next day in order to finish.  Whaaaatt?  Yes, my little kiddos were figuring out how many stripes we needed in order for every student in the class to make an American flag.  How man red stripes, how many white, and how many in total.  Yes, first graders were working on this problem.  I figure if they can add 7+7 to figure out how many stripes on two flags, why not three, or seven, or sixteen?  And they did it!  Even better, they did it with a sense of excitement and enthusiasm.

Some used blocks, made piles, rearranged them into groups of tens and counted.  Some drew arrays of squares, colored in groups of ten and counted that way.  Some used multiplication to figure out the number of red stripes, then subtracted sixteen to find the number of white stripes.  And here's the kicker... I refused to check their answers.  I'm training these kids to be mathematicians, not to fill out a worksheet.  How often in your adult life do you fill out worksheets?  This means the kiddos have to find an answer, and solve the problem in at least one more way where they will hopefully get the same answer.  Then they need to check their work against that of fellow mathematicians (classmates) to see if the results can be replicated.  This is how mathematicians work in the real world, so this is how the mathematicians in my class work.  We call this comparing of strategies and answers Math Conferences.  I go to teacher math conferences to learn from and teach other teachers, so my students go to student math conferences to learn from and teach other students.

Now this work ethic didn't magically implant itself in the brains of my students, we trained for this.  We do work on daily math lessons with instruction and practice, and we start the year with simple math problems.  But just like training for a marathon, you build strength and stamina until you are a finely trained athlete.  The same is true for becoming a mathematician.  All it takes is training, practice, and perseverance.  Working persistently, and for great lengths of time, in order to solve something that needs to be solved is a skill that will serve these children well in their lives.  Careers, relationships, life traumas and emergencies... all of these require hard work, not giving up, and finding that solution that works.  So, I hope that my little mathematicians, unlike most Americans, will retain and foster these skills, and grow up to say, "I AM a math person."